campusgugl.blogg.se

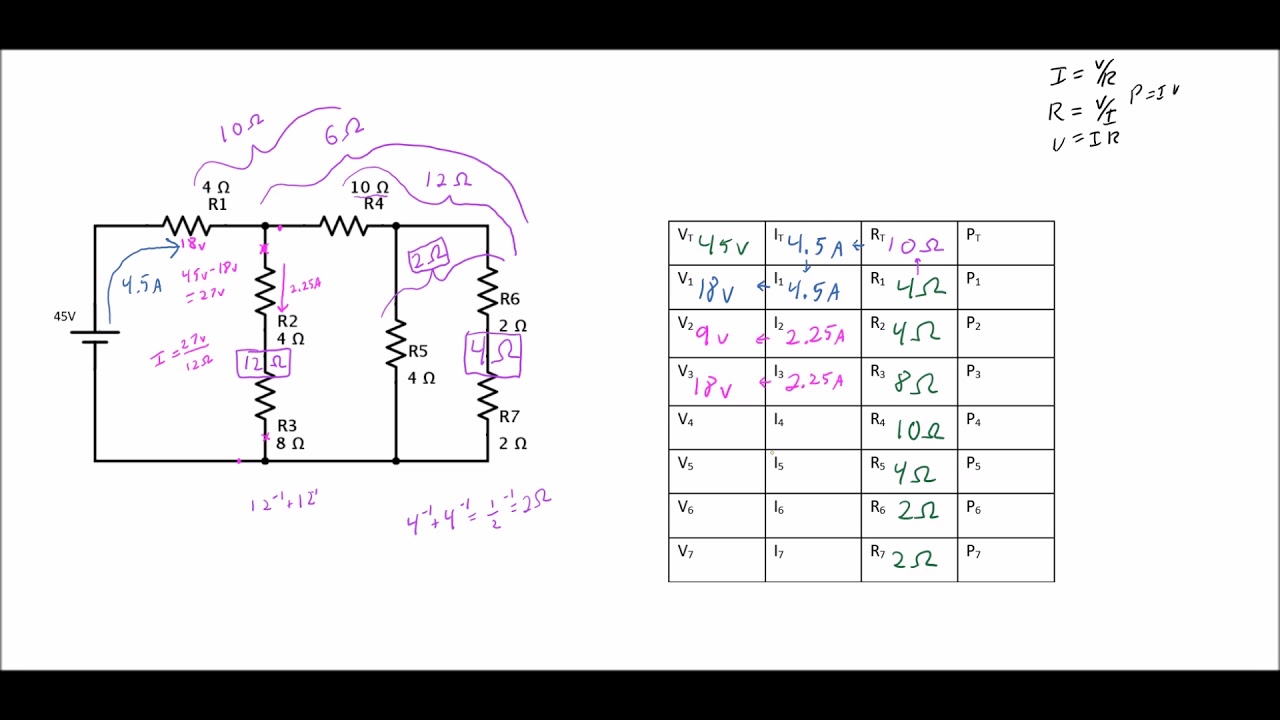
Learning new words is an essential aspect of human language, which is not limited to childhood as adults also add new words to their mental lexicon. In conclusion, our study confirms that cTBS can induce lasting modulations of neural processes which are associated with learning, but the effect depends on the individual network state. Only participants with high intrinsic alpha-band coherence between the stimulated area and the rest of the brain before stimulation showed the expected inhibition during naming and greater learning performance. Behavioral and neural responses to cTBS were variable across participants and were associated with the magnitude of resting-state alpha-band coherence between the stimulated area and the rest of the brain before stimulation. Inhibition of the right Broca homolog did not affect new word learning performance at the group level. Neural effects were assessed with electroencephalography (EEG) source power analyses during the naming task as well as coherence analyses at rest 1 day before and after training. Sixteen young participants trained a new-word learning paradigm with rare, mostly unknown objects and their corresponding words immediately after continuous theta burst stimulation (cTBS) or sham stimulation of right inferior frontal gyrus (IFG) in a cross-over design. This study examined whether an inhibition of the right Broca homolog has long-term impact on neural processes underlying the acquisition of new words in healthy individuals. However, previous studies have mostly assessed short-term effects of rTMS on language performance and the effect on learning is largely unknown. It would be important to obtain a similar enhancement for verbal learning. Repetitive transcranial magnetic stimulation (rTMS) has demonstrated beneficial effects on motor learning. 3Faculty of Psychology and Educational Sciences, University of Geneva, Geneva, Switzerland.2Laboratory of Cognitive Neurorehabilitation, Department of Clinical Neurosciences, Medical School, University of Geneva, Geneva, Switzerland.1Division of Neurorehabilitation, Department of Clinical Neurosciences, University Hospital Geneva, Geneva, Switzerland.Pacific Grove, CA: Brooks/Cole Publishing Company, 2001.Pierre Nicolo 1,2, Raphaël Fargier 3, Marina Laganaro 3 and Adrian G. Discrete Mathematics and Its Applications, 3rd ed. London, U.K.: Jones and Bartlett Publishers, 1996. Thus, the fifth power of the sum of a and b, ( a + b ) 5, would be expressed as. For convenience of notation, C ( n, r ) is often expressed as. The number of different combinations available, since putting toppings on a pizza is not an ordered collection, is C (7,0) + C (7,1) + C (7,2) + C (7,3) + C (7,4) + C (7,5) + C (7,6) + C (7,7).

Another application of combinations might be to suppose that a local pizza parlor has seven different toppings available, besides cheese, which is on every pizza. This situations suggests that the number of combinations with r objects, r -combinations, from a collection with n objects, C ( n, r ), is,, and this formula suggests. Initially one would know that there are permutations, but 3! permutations represent a combination, so there are × combinations. An extended example would be to consider 100 distinct points on a circle and to inquire as to number of unique trigons, a combination, which would be determined with the vertices. In how many different ways could these candidates be elected to the three offices? One way to find out is to simply list the possible permutations.

Suppose a club has two candidates for president: Bob (B) and Janice (J) three candidates for secretary: Katy (K), Rob (R), and Harry (H) and three candidates for parliamentarian: Abe (A), Calvin (C), and Mary (M). Sometimes one is interested in knowing the number of permutations that are available from a collection of objects. The ordered arrangements of objects likely dates all the way to the beginning of organizing and recording information. For instance, the fraction is a permutation of two objects, whereas the combination to open a lock -23 L, 5 R, and 17 L -is a permutation of three objects. PermutationsĪ permutation is an ordered arrangement of objects.

The study of permutations and combinations is at the root of several topics in mathematics such as number theory, algebra, geometry, probability, statistics, discrete mathematics, graph theory, and many other specialties.